"Exponentiation is used extensively in many fields, including economics, biology, chemistry, physics, and computer science, with applications such as compound interest, population growth, chemical reaction kinetics, wave behavior, and public-key cryptography."
Advertisement:
Read Later
"Exponentiation is used extensively in many fields, including economics, biology, chemistry, physics, and computer science, with applications such as compound interest, population growth, chemical reaction kinetics, wave behavior, and public-key cryptography."
Related Links :
Exponentiation"Exponentiation is used extensively in many fields, including economics, biology, chemistry, physics, and computer science, with applications such as compound interest, population growth, chemical reaction kinetics, wave behavior, and public-key cryptography."
, Symbol for Integer;
, Symbol for Integer;
, Symbol for Integer;
Just as a repeated sum a + a + ⋯ + a of k summands is written ka, so a repeated product a × a × ⋯ × a of k factors is written ak. The number k is called the exponent, and a the base of the power ak. At this point an interesting development occurs, for, so long as only additions and multiplications are performed with integers, the resulting numbers are invariably themselves integers—that is, numbers of the same kind as their antecedents. This characteristic changes drastically, however, as soon as division is introduced. Performing division (its symbol ÷, read “divided by”) leads to results, called quotients or fractions, which surprisingly include numbers of a new kind—namely, rationals—that are not integers. These, though arising from the combination of integers, patently constitute a distinct extension of the natural-number and integer concepts as defined above. By means of the application of the division operation, the domain of the natural numbers becomes extended and enriched immeasurably beyond the integers.
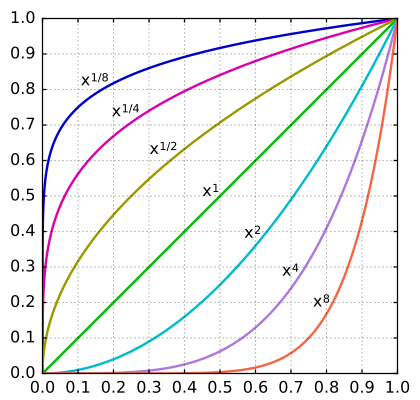
Figure - 11.1 By Geek3 - Own work, CC BY-SA 4.0, https://commons.wikimedia.org/w/index.php?curid=64336014